Giải bài 3.34 trang 49 sách bài tập toán 7 - Kết nối tri thức với cuộc sống - Ôn tập chương 3

Cho hình 3.34. Biết AB∥Cx;ˆA=700;ˆB=600.AB∥Cx;ˆA=700;ˆB=600.
Tính số đo các góc ^C1;^C2;^C3.ˆC1;ˆC2;ˆC3.
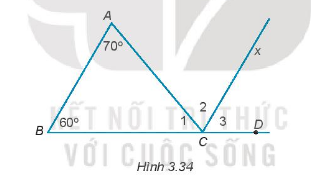

- Tính ^C2ˆC2
- Tính ^C3ˆC3
Advertisements (Quảng cáo)
- Tính ^C1ˆC1

Vì AB//Cx⇒^BAC=^C2AB//Cx⇒ˆBAC=ˆC2 (2 góc so le trong). Mà ^BAC=700ˆBAC=700 nên ^C2=700ˆC2=700
^ABC=^C3ˆABC=ˆC3 (2 góc đồng vị). Mà ^ABC=600ˆABC=600 nên ^C3=600ˆC3=600
Mặt khác,^C1+^ACD=1800ˆC1+ˆACD=1800 (2 góc kề bù)
⇒^C1+(^C2+^C3)=1800⇒^C1+(700+600)=1800⇒^C1+1300=1800⇒^C1=1800−1300⇒^C1=500
