Câu hỏi/bài tập:
Cho biểu thức \(P = \frac{2}{{\sqrt x - 1}} + \frac{2}{{\sqrt x + 1}} - \frac{{5 - \sqrt x }}{{x - 1}}\) với \(x \ge 0,x \ne 1\).
a) Rút gọn biểu thức P.
b) Tìm giá trị của P tại \(x = 1\).
c) Tìm giá trị của \(x\) để P nguyên.
a) Quy đồng các phân thức.
b) Thay\(x = 1\) và biểu thức P đã rút gọn.
c) Chặn 2 đầu của P:
Với \(x \ne 1,x \ge 0\) ta có \(\sqrt x + 1 \ge 1\) nên \(\frac{5}{{\sqrt x + 1}} \ge 0\) và \(\frac{5}{{\sqrt x + 1}} \le 5\). Do đó \(0 < P \le 5\).
Advertisements (Quảng cáo)
a) Điều kiện xác định: \(x \ne 1,x \ge 0\)
\(\begin{array}{l}P = \frac{2}{{\sqrt x - 1}} + \frac{2}{{\sqrt x + 1}} - \frac{{5 - \sqrt x }}{{x - 1}}\\ = \frac{{2\left( {\sqrt x + 1} \right)}}{{\left( {\sqrt x - 1} \right)\left( {\sqrt x + 1} \right)}} + \frac{{2\left( {\sqrt x - 1} \right)}}{{\left( {\sqrt x + 1} \right)\left( {\sqrt x - 1} \right)}} - \frac{{5 - \sqrt x }}{{\left( {\sqrt x + 1} \right)\left( {\sqrt x - 1} \right)}}\\ = \frac{{2\left( {\sqrt x + 1} \right) + 2\left( {\sqrt x - 1} \right) - \left( {5 - \sqrt x } \right)}}{{\left( {\sqrt x - 1} \right)\left( {\sqrt x + 1} \right)}}\\ = \frac{{2\sqrt x + 2 + 2\sqrt x - 2 - 5 + \sqrt x }}{{\left( {\sqrt x - 1} \right)\left( {\sqrt x + 1} \right)}}\\ = \frac{{5\sqrt x - 5}}{{\left( {\sqrt x - 1} \right)\left( {\sqrt x + 1} \right)}}\\ = \frac{{5\left( {\sqrt x - 1} \right)}}{{\left( {\sqrt x - 1} \right)\left( {\sqrt x + 1} \right)}} = \frac{5}{{\sqrt x + 1}}\end{array}\)
b) Thay \(x = 1\) (TMĐK) vào P, ta được \(P = \frac{5}{{\sqrt x + 1}} = \frac{5}{{\sqrt 1 + 1}} = \frac{5}{2}\)
Vậy \(P = \frac{5}{2}\) khi \(x = 1\).
c) Với \(x \ne 1,x \ge 0\) ta có \(\sqrt x + 1 \ge 1\) nên \(\frac{5}{{\sqrt x + 1}} \ge 0\) và \(\frac{5}{{\sqrt x + 1}} \le 5\). Do đó \(0 < P \le 5\).
Vậy để P nguyên thì \(P \in \left\{ {1;2;3;4;5} \right\}\).
Ta có bảng sau:
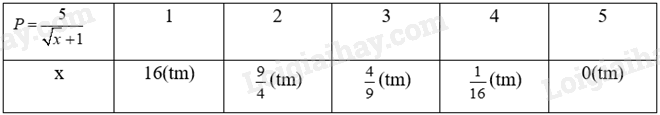
Vậy \(x \in \left\{ {16;\frac{9}{4};\frac{4}{9};\frac{1}{{16}};0} \right\}\) là các giá trị cần tìm.