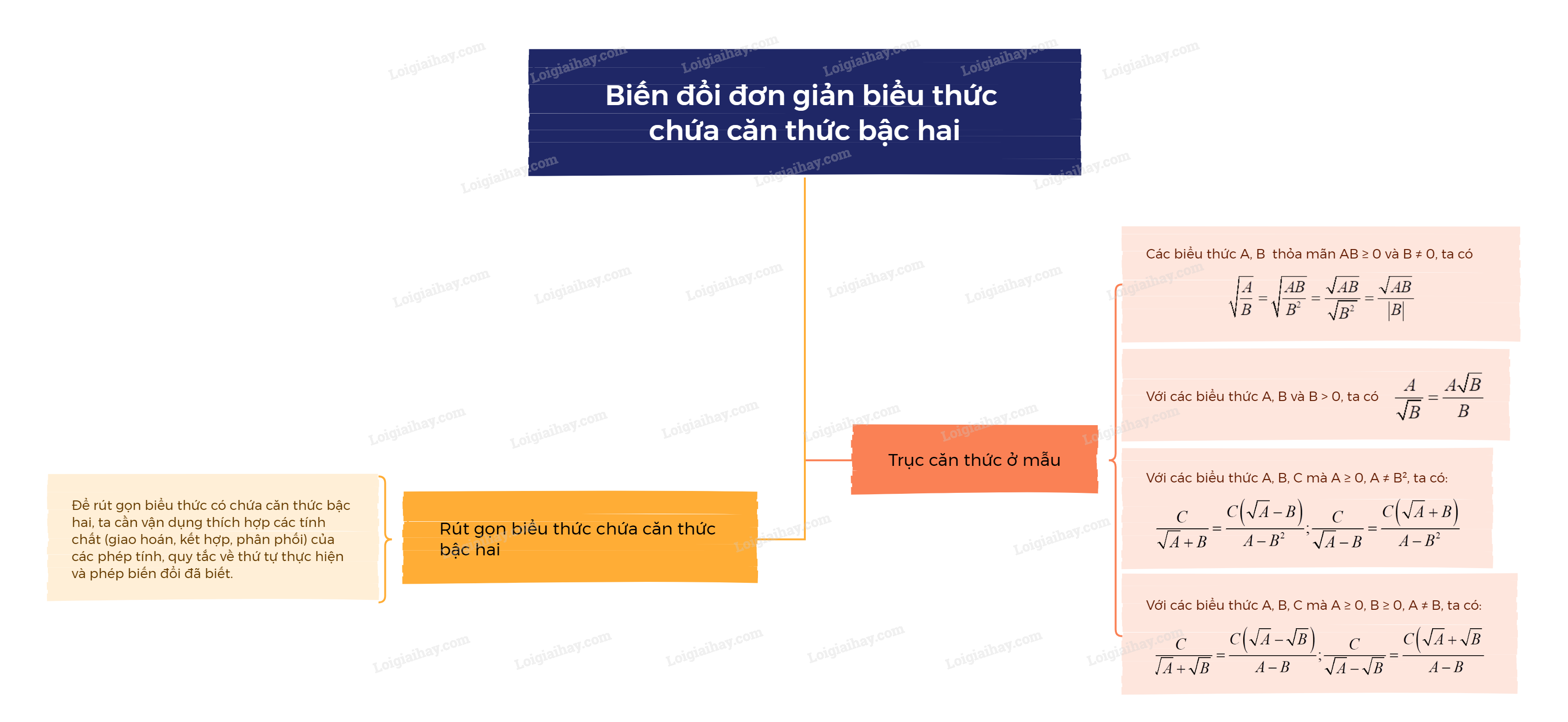
1. Trục căn thức ở mẫu
|
- Với các biểu thức A và B thỏa mãn \(AB \ge 0,B \ne 0\), ta có: \(\sqrt {\frac{A}{B}} = \sqrt {\frac{{AB}}{{{B^2}}}} = \frac{{\sqrt {AB} }}{{\sqrt {{B^2}} }} = \frac{{\sqrt {AB} }}{{\left| B \right|}}\). - Với các biểu thức A, B và B > 0, ta có \(\frac{A}{{\sqrt B }} = \frac{{A\sqrt B }}{B}\). - Với các biểu thức A, B, C mà \(A \ge 0,A \ne {B^2}\), ta có: \(\frac{C}{{\sqrt A + B}} = \frac{{C\left( {\sqrt A - B} \right)}}{{A - {B^2}}};\frac{C}{{\sqrt A - B}} = \frac{{C\left( {\sqrt A + B} \right)}}{{A - {B^2}}}\). - Với các biểu thức A, B, C mà \(A \ge 0,B \ge 0,A \ne B\), ta có: \(\frac{C}{{\sqrt A + \sqrt B }} = \frac{{C\left( {\sqrt A - \sqrt B } \right)}}{{A - B}};\frac{C}{{\sqrt A - \sqrt B }} = \frac{{C\left( {\sqrt A + \sqrt B } \right)}}{{A - B}}\). |
Ví dụ:
Advertisements (Quảng cáo)
\(\frac{2}{{3\sqrt 5 }} = \frac{{2\sqrt 5 }}{{3{{\left( {\sqrt 5 } \right)}^2}}} = \frac{{2\sqrt 5 }}{{3.5}} = \frac{{2\sqrt 5 }}{{15}}\);
\(\frac{a}{{3 - 2\sqrt 2 }} = \frac{{a\left( {3 + 2\sqrt 2 } \right)}}{{\left( {3 - 2\sqrt 2 } \right).\left( {3 + 2\sqrt 2 } \right)}} = \frac{{a\left( {3 + 2\sqrt 2 } \right)}}{{{3^2} - {{\left( {2\sqrt 2 } \right)}^2}}} = \frac{{a\left( {3 + 2\sqrt 2 } \right)}}{{9 - 8}} = \left( {3 + 2\sqrt 2 } \right)a\).
2. Rút gọn biểu thức chứa căn thức bậc hai
|
Để rút gọn biểu thức có chứa căn thức bậc hai, ta cần vận dụng thích hợp các tính chất (giao hoán, kết hợp, phân phối) của các phép tính, quy tắc về thứ tự thực hiện và phép biến đổi đã biết. |
Ví dụ:
\(\begin{array}{l}A = 2\sqrt 3 - \sqrt {75} + \sqrt {{{\left( {1 - \sqrt 3 } \right)}^2}} \\ = 2\sqrt 3 - \sqrt {{{3.5}^2}} + \left| {1 - \sqrt 3 } \right|\\ = 2\sqrt 3 - 5\sqrt 3 + \sqrt 3 - 1\\ = - 1 - 2\sqrt 3 \end{array}\)
\(\begin{array}{l}B = x\sqrt x - \frac{{{x^2} - x}}{{\sqrt x + 1}}\\ = x\sqrt x - \frac{{\left( {{x^2} - x} \right)\left( {\sqrt x - 1} \right)}}{{\left( {\sqrt x + 1} \right)\left( {\sqrt x - 1} \right)}}\\ = x\sqrt x - \frac{{x\left( {x - 1} \right)\left( {\sqrt x - 1} \right)}}{{\left( {\sqrt x + 1} \right)\left( {\sqrt x - 1} \right)}}\\ = x\sqrt x - \frac{{x\left( {x - 1} \right)\left( {\sqrt x - 1} \right)}}{{x - 1}}\\ = x\sqrt x - x\left( {\sqrt x - 1} \right)\\ = x\sqrt x - x\sqrt x + x\\ = x\end{array}\)
