Giải bài 4.60 trang 70 sách bài tập toán 10 - Kết nối tri thức với cuộc sống - Bài tập cuối chương IV : Trên cạnh BC của tam giác ABC lấy các điểm M,N, không trùng với B và C sao cho BM=MN=NC. .Chứng minh rằng hai tam giác ABC và AMN có cùng trọng tâm...

Trên cạnh BC của tam giác ABC lấy các điểm M,N, không trùng với B và C sao cho BM=MN=NC.
a) Chứng minh rằng hai tam giác ABC và AMN có cùng trọng tâm.
b) Gọi G là trọng tâm của tam giác ABC. Đặt →GB=→u và →GC=→v. hãy biểu thị các vectơ sau qua hai vectơ →u và →v:→GA,→GM,→GN.

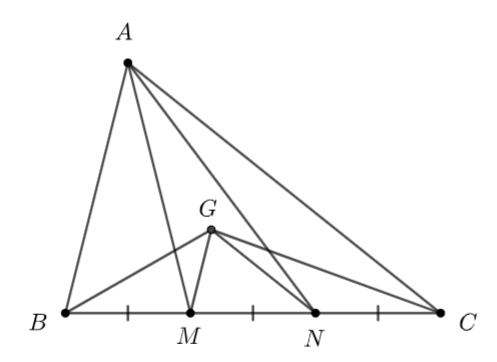
a) Ta có: →AA+→BM+→CN=→BM+→CN=→0
⇒ hai tam giác ABC và AMN có cùng trọng tâm.
b) Gọi G là trọng tâm của tam giác ABC. Đặt →GB=→u và →GC=→v.
Advertisements (Quảng cáo)
Ta có: G là trọng tâm của tam giác ABC.
⇒ →GA+→GB+→GC=→0
⇒ →GA=−→GB−→GC=−→u−→v=−(→u+→v)
Ta có: →GM=→GB+→BM
=→GB+13→BC
=→GB+13(→GC−→GB)=23→GB+13→GC=13(2→u+→v)
Ta có: →GN=→GC+→CN
=→GC+13→CB=→GC+13(→GB−→GC)=13→GB+23→GC=13(→u+2→v)
