Cho tứ diện ABCD trong đó \(AB \bot AC,AB \bot B{\rm{D}}\). Gọi P và Q lần lượt là trung điểm của AB và CD. Chứng minh rằng AB và PQ vuông góc với nhau.

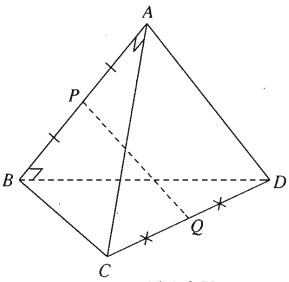
\(\eqalign{
& \overrightarrow {PQ} = \overrightarrow {PA} + \overrightarrow {AC} + \overrightarrow {CQ} \,\,\,\,\,\,\,\,\,\,\,\left( 1 \right) \cr
& \overrightarrow {PQ} = \overrightarrow {PB} + \overrightarrow {B{\rm{D}}} + \overrightarrow {DQ} \,\,\,\,\,\,\,\,\,\,\left( 2 \right) \cr} \)
Advertisements (Quảng cáo)
Cộng từng vế (1) và (2) ta có:
\(2\overrightarrow {PQ} = \overrightarrow {AC} + \overrightarrow {B{\rm{D}}} \)
Suy ra \(2\overrightarrow {PQ} .\overrightarrow {AB} = \overrightarrow {AC} .\overrightarrow {AB} + \overrightarrow {B{\rm{D}}} .\overrightarrow {AB} = 0\)
Hay \(\overrightarrow {PQ} .\overrightarrow {AB} = 0\), tức là \(PQ \bot AB\).
