Bài tập 1 trang 156 Tài liệu dạy – học Toán 7 tập 1, Ở hình 53 cho biết...

Ở hình 53 cho biết $AD = BC,\,\,\widehat {ADC} = \widehat {BCD}.$ Chứng minh rằng:

$\eqalign{  & a)\,\,\Delta ADC = \Delta BCD  \cr  & b)IA = IB \cr}$

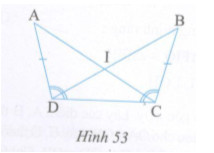
a)Xét tam giác ADC và BCD ta có:

AD = BC (gt)

$\widehat {ADC} = \widehat {BCD}(gt)$

DC là cạnh chung.

Do đó: $\Delta ADC = \Delta BCD(c.g.c)$

b) Ta có:

$\eqalign{  & *\Delta ADC = \Delta BCD \Rightarrow \widehat {DAC} = \widehat {CBD};\widehat {ACD} = \widehat {BDC}  \cr  & \widehat {ADI} + \widehat {IDC} = \widehat {ADC};\widehat {BCI} + \widehat {ICD} = \widehat {BCD} \cr}$

Mà $\widehat {ADC} = \widehat {BCD};\widehat {ACD} = \widehat {BDC}$  nên $\widehat {ADI} = \widehat {BCI}$

Xét tam giác ADI và BCI ta có: $\eqalign{  & \widehat {ADI} = \widehat {BCI}(cmt)  \cr  & AD = BC(gt)  \cr  & \widehat {DAI} = \widehat {CBI}(\widehat {DAC} = \widehat {CBD}) \cr}$

Do đó: $\Delta ADI = \Delta BCI(g.c.g) \Rightarrow IA = IB.$