Tam giác \(ABC\) có \(\widehat B = {60^0}; \widehat C = {45^0}; BC = a\).
a) Tính độ dài hai cạnh \(AB, AC.\)
b) Chứng minh \(\cos {75^0} = \dfrac{{\sqrt 6 - \sqrt 2 }}{4}\).
Giải
a) Ta có \(\widehat A = {180^0} - ({60^0} + {45^0}) = {75^0}.\)
Advertisements (Quảng cáo)
Đặt \(AC=b, AB=c\). Theo định lí hàm sớ sin:
\(\dfrac{b}{{\sin {{60}^o}}} = \dfrac{a}{{\sin {{75}^0}}} = \dfrac{c}{{{\mathop{\rm s}\nolimits} {\rm{in4}}{5^0}}}\).
Suy ra \(b = \dfrac{{a\sqrt 3 }}{{2\sin {{75}^0}}} ; c = \dfrac{{a\sqrt 2 }}{{2\sin {{75}^0}}}.\)
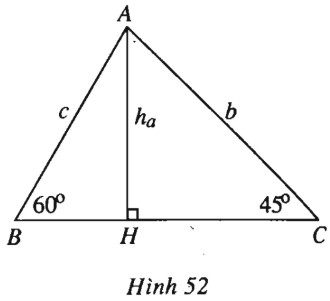
b) Kẻ \(AH \bot BC\) (h.52), do \(\widehat B, \widehat C\) đều là góc nhọn nên \(H\) thuộc đoạn \(BC\), hay \(BC=HB+HC\). Ta có
\(\begin{array}{l}\left\{ \begin{array}{l}HC = \dfrac{{b\sqrt 2 }}{2}\\HB = \dfrac{c}{2}\end{array} \right.\\ \Rightarrow a = HC + HB = b\dfrac{{\sqrt 2 }}{2} + \dfrac{c}{2} \\= \dfrac{{a\sqrt 6 + a\sqrt 2 }}{{4.\sin {{75}^0}}} \\ \Rightarrow \sin {75^0} = \dfrac{{\sqrt 6 + \sqrt 2 }}{4}.\\\cos {75^0} = \sqrt {1 - {{\sin }^2}{{75}^0}}\\ = \sqrt {1 - {{\left( {\dfrac{{\sqrt 6 + \sqrt 2 }}{4}} \right)}^2}} \\ = \dfrac{1}{4}\sqrt {8 - 2\sqrt {12} } \\ = \dfrac{1}{4}\sqrt {{{\left( {\sqrt 6 - \sqrt 2 } \right)}^2}} = \dfrac{{\sqrt 6 - \sqrt 2 }}{4}\end{array}\)
