Bài tập 1 trang 103 Tài liệu dạy – học Toán 8 tập 1, Tính các góc của tứ giác trong hình 36:...

Tính các góc của tứ giác trong hình 36:

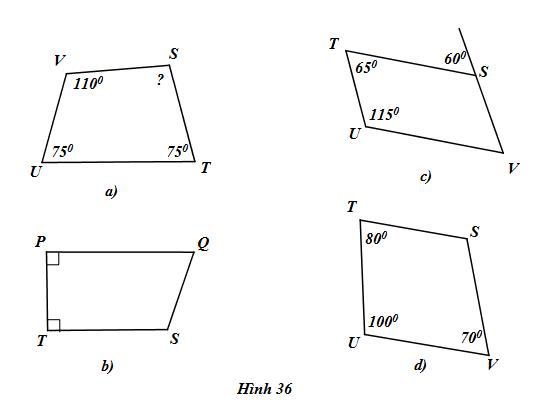
a) Tứ giác UVST có: $\widehat U + \widehat V + \widehat T + \widehat S = {360^0}$

Do đó: ${75^0} + {110^0} + {75^0} + \widehat S = {360^0}$

$\Rightarrow \widehat S = {360^0} - \left( {{{75}^0} + {{110}^0} + {{75}^0}} \right) = {360^0} - {260^0} = {100^0}$

b) Tứ giác TPQS có  $\widehat T + \widehat P + \widehat Q + \widehat S = {360^0}$

Do đó: ${90^0} + {90^0} + {70^0} + \widehat S = {360^0}$

$\Rightarrow \widehat S = {360^0} - \left( {{{90}^0} + {{90}^0} + {{70}^0}} \right) = {360^0} - {250^0} = {110^0}$

c) Tứ giác TUVS có: $\widehat T + \widehat U + \widehat V + \widehat S = {360^0}$

Do đó: ${65^0} + {115^0} + {60^0} + \widehat S = {360^0}$

$\Rightarrow \widehat S = {360^0} - \left( {{{65}^0} + {{115}^0} + {{60}^0}} \right) = {360^0} - {240^0} = {120^0}$

d) Tứ giác TURS có: $\widehat T + \widehat U + \widehat R + \widehat S = {360^0}$

Do đó: ${80^0} + {100^0} + {70^0} + \widehat S = {360^0}$

$\Rightarrow \widehat S = {360^0} - \left( {{{80}^0} + {{100}^0} + {{70}^0}} \right) = {360^0} - {240^0} = {110^0}$